Learning mathematics, while interesting and rewarding, also tends to be challenging for students just starting their studies. What makes learning mathematics so challenging? In this blog post, we will look at learning mathematics from the aspect of the information processing theory, specifically the cognitive load theory.
The information processing theory explains how we process information while using three types of memory storage: short-term memory, working memory, and long-term memory (Sweller et al., 1956).
We get stimuli from our surroundings and register these in our short-term memory. When we pay attention to certain stimuli, those can go further down the funnel and arrive at our working memory storage. Here, we can keep the information for a short period of time, as long as we need it. After that, the information will be lost unless we put conscious effort into remembering it, in which case the information will be stored in the long-term memory. According to Kirschner et al. (2010, p75), “learning is defined as a change in long-term memory”.
Let’s illustrate these concepts with an example. While sitting in class, a student might hear the sound of the other students moving around or perhaps feel a slight breeze coming from the open window. These are both stimuli from the environment that enter short-term memory. The voice of the teacher is also one of the stimuli coming to the student from their surroundings. When the student pays attention to this voice, it enters the student’s working memory. From here, the student can process the incoming information by associating it with the previous knowledge. If the teacher is talking about calculating the surface area of a rectangle, the student might remember how their parents were calculating the surface of a room in order to determine how much paint they would need for the walls. The new information gets included in the student’s knowledge by being stored in the long-term memory.
This process of information processing is essential to understanding how we learn, specifically mathematics. The working memory is the critical spot of this process. It’s the decision point where the information can be saved or lost.
Sweller et al. (1998, p252) state that “working memory can be equated with consciousness.” Working memory is the space for us to handle information, whether that is remembering a phone number as long as we need to type it into the phone or recalling an address to write on a letter.
Research shows that our working memory is significantly limited in its capacity and duration (Miller, 1956). We can only keep a limited number of elements of information (+-7) and only for a very short period of time.
Based on the research done in the domain of working memory came the cognitive load theory, which focuses on the requests posed to our working memory. Sweller et al. (1998) identify three types of cognitive load: intrinsic, extrinsic, and germane.

Intrinsic cognitive load is the load that comes from the material we are learning. This load comes from the complexity of the material itself, for example, if we are learning a new complex theory of mathematics.
On the other hand, extrinsic cognitive load includes any other load, excluding the material itself. This load might include the way that the material is presented, the type of presentation we used, and the conditions in the room where we are learning.
Germane load balances the first two. “For instance, consciously connecting new information with what is already known, rather than focusing on task details is a process that yields germane cognitive load” (Kirschner et al., 2009, p207).
While teachers often don’t have much influence on the intrinsic cognitive load, they can relieve some of the extrinsic cognitive load. In this blog post, we will focus on the tools that teachers can use to reduce the extrinsic cognitive load.
Van Merriënboer et al. (2003) mention scaffolding as a means of reducing the cognitive load. As they propose (2003, p4), using the scaffolding technique, the teacher initially provides significant support to students as they get started with a new topic: “coaching by providing hints, prompts, and feedback; modeling the use of cognitive strategies by thinking aloud; presenting cue cards, checklists and process worksheets; asking leading questions, and giving part of a solution are all examples of such support”. Over time, the support is slowly removed, and the student learns to solve the problem on their own.
During a mathematics lesson, a teacher might ask questions such as: what are you trying to solve? Have you seen this problem before? How do you know that is the answer?
These questions allow the student to lead the learning process instead of the teacher.
Problem-based learning has been examined as another tool to reduce the cognitive load. As defined by Roh ( 2003, p2),
“problem-Based Learning describes a learning environment where problems drive the learning. That is, learning begins with a problem to be solved, and the problem is posed in such a way that students need to gain new knowledge before they can solve the problem”.
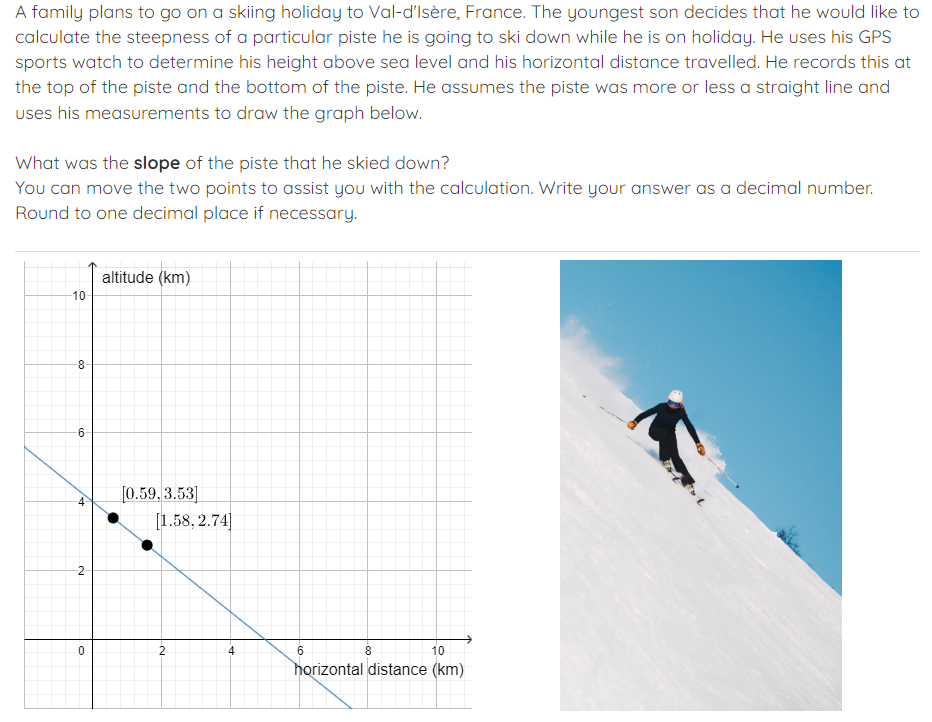
A study by Tarmizi & Bayat (2012) examined the effects of problem-based learning on an educational statistics course. After a 10-week course consisting of scenarios and guided questions, they compared the student performance of a problem-based learning group and a group who didn’t use the problem-based approach. There was a significant difference between the mean performance of the problem-based learning group and that of the conventional group, indicating problem-based learning efficacy (Tarmizi & Bayat, 2012, p344).
Sowiso is an online tool for learning STEM subjects. The ready-made courses include exercises and theory pages, and some include videos as well.
Theory pages are well organized to show only the necessary information to avoid overwhelming a student who is new to the subject. Additional information is hidden under slots, offering definitions, examples, and other resources.
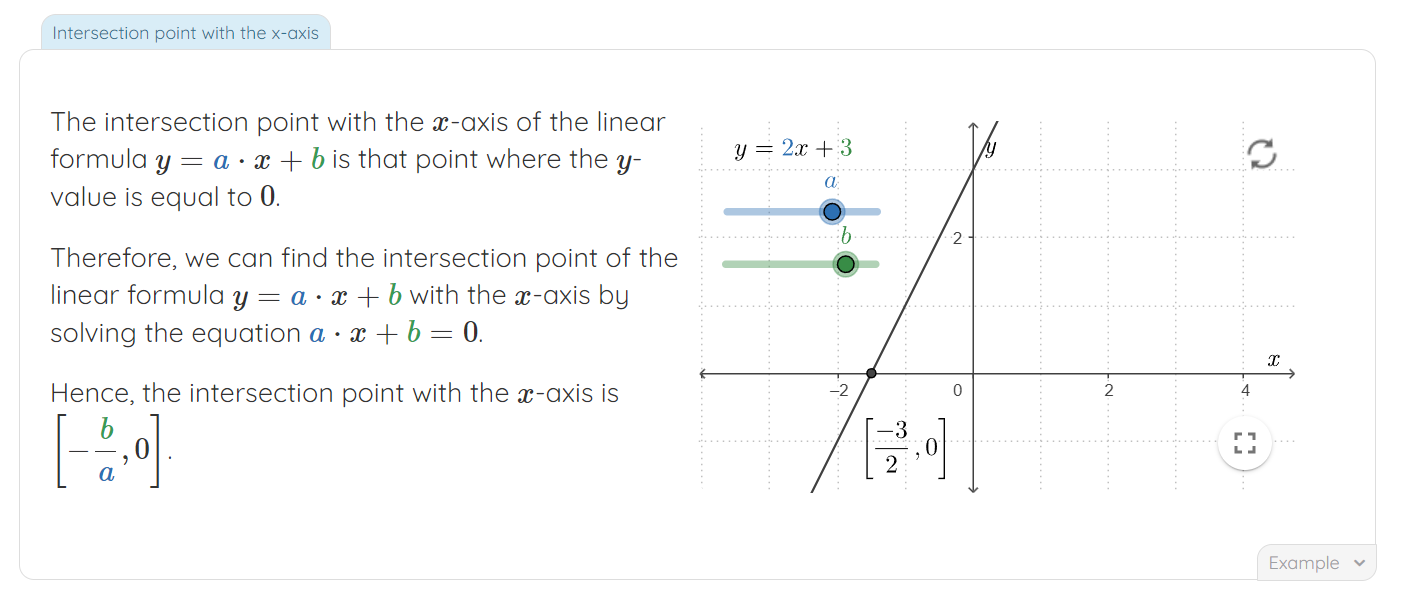
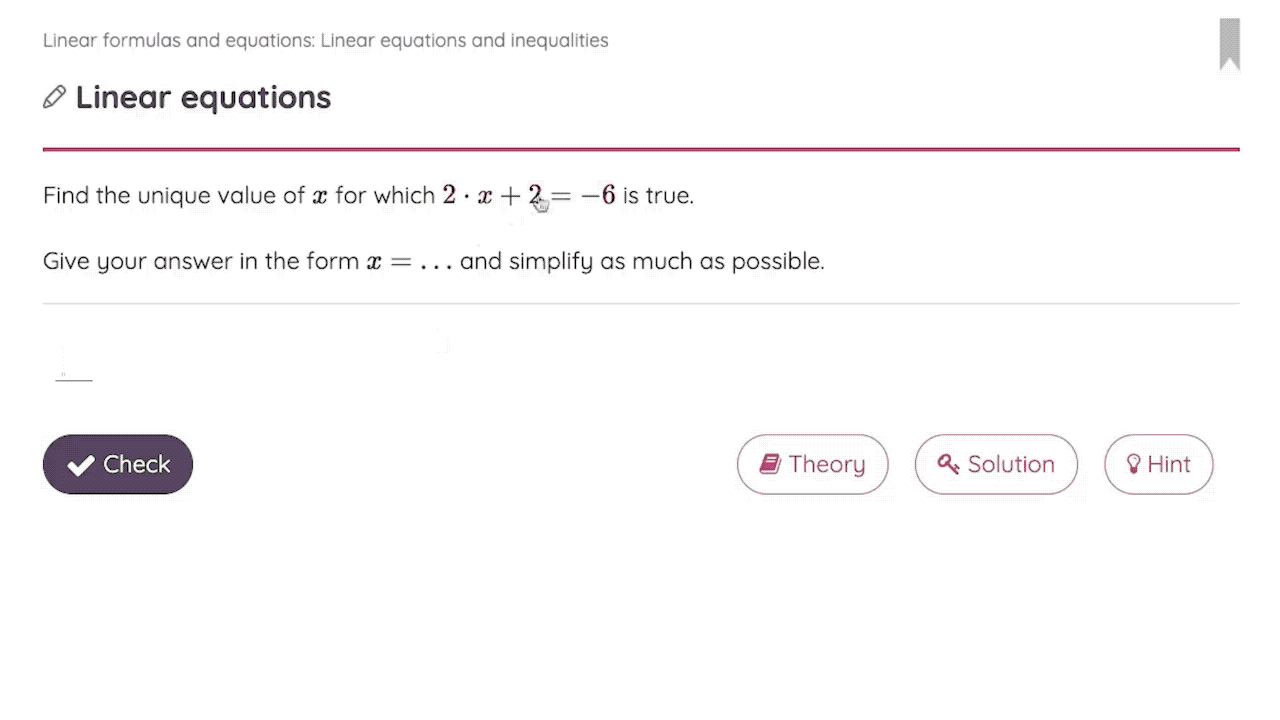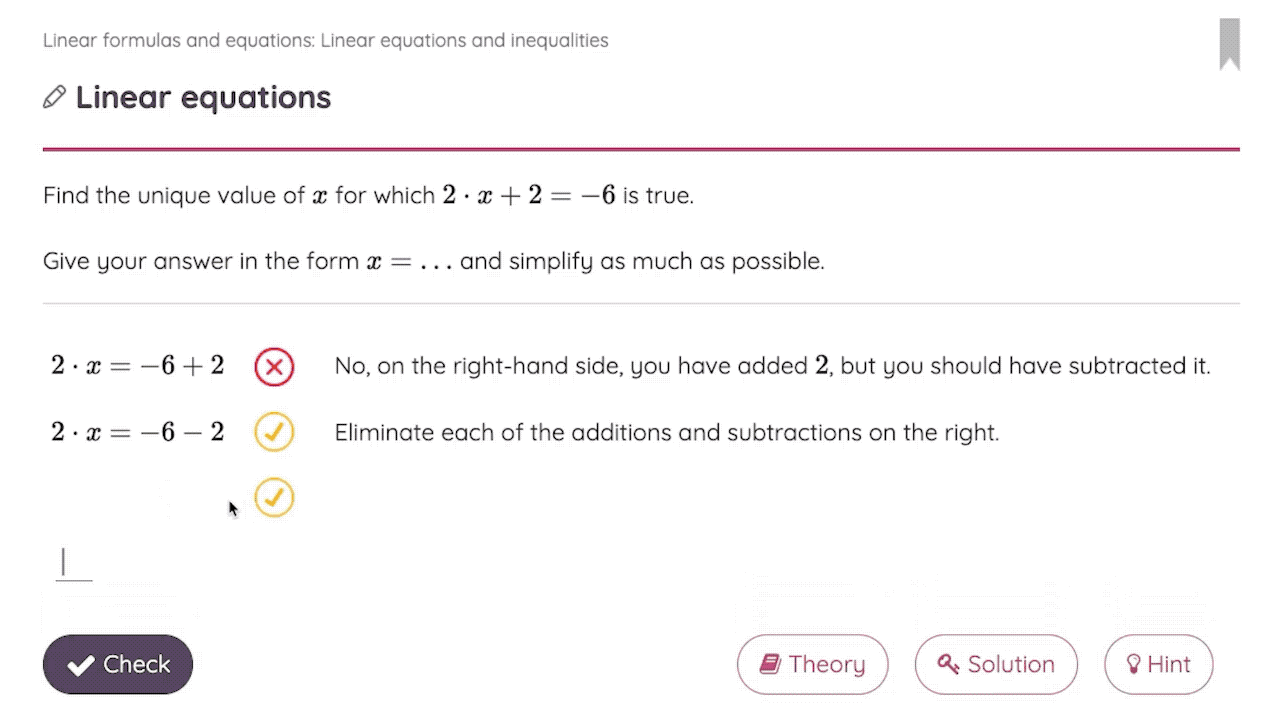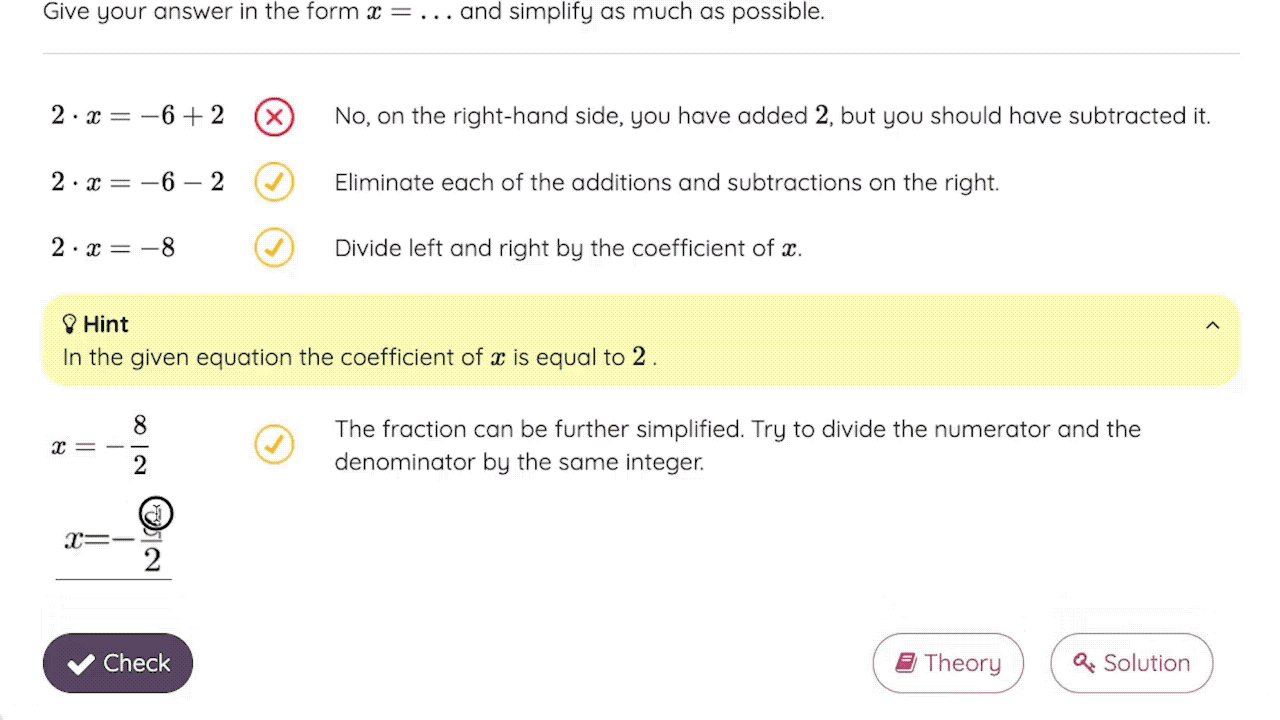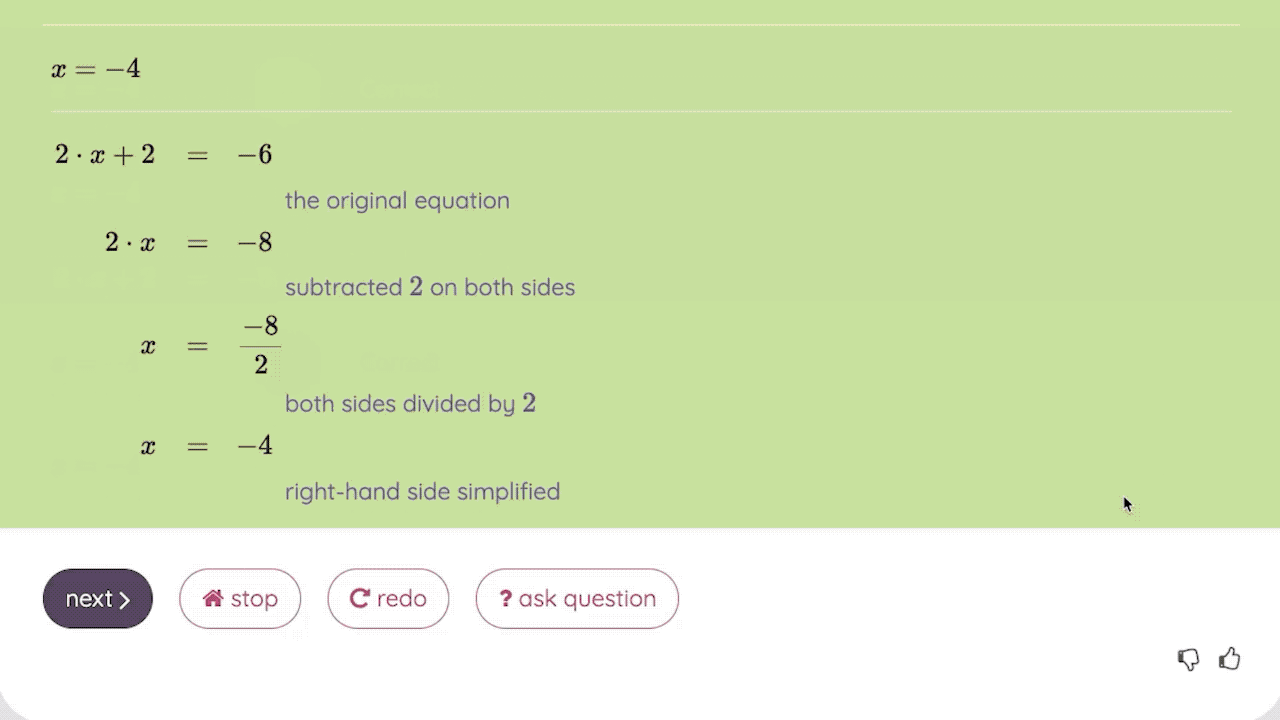
Sowiso offers unlimited practice, providing hints and worked-out solutions. These are great for providing scaffolding without requiring more teacher engagement.

Learn how to use preparatory math courses to ensure all students have the required mathematical proficiency before starting the lessons.

Using digital technology to implement learning scaffolds that better support students in large heterogeneous math and stats classes at universities and colleges.

Learn what personalized learning in mathematics is and its benefits. Discover how technology facilitates this student-centered approach.